Bertrand Russell Proof 1 1 2

Peano shows that it s not hard to produce a useful set of axioms that can prove 1 1 2 much more easily than whitehead and russell do.

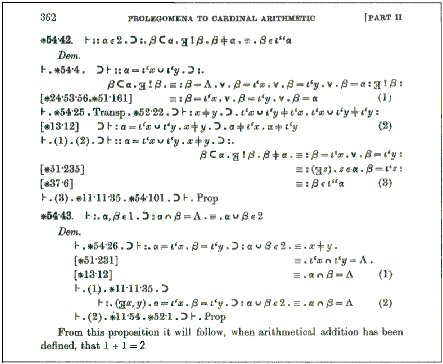
Bertrand russell proof 1 1 2. The main reason that it takes so long to get to 1 1 2 is that principia mathematica starts from almost nothing and works its way up in very tiny incremental steps. Here on page 362 they finally get around to proving that 1 1 2. Wasn t bertrand s proof thorough enough. This certainly isn t the longest proof that 1 1 2.

A proof of that statement and as such a logical system for all arithmetic was one of the life long goals of logicians bertrand russell and alfred north whitehead. In it they laid the foundation of modern mathematics. In order to prove mathematics from the very beginning you have to establish the first principle of arithmetic and that piece of symbolic logic was proving that one plus one equals two. After all we have had many centuries if not m.

What is difficult is defining 1 2 and. Then another 20 pages before he defined 1 1 2. The work of g. On bertrand russell s proof that 1 1 2 stephen fry.

To be fair this wasn t the main point of their effort the peano ax. No one with a clear mind will deny that this equation holds true in practice. Then it was close to the end of the book before he finally proved it. We ll just take their word for it.

That honour probably goes to alfred north whitehead and bertrand russell in principia mathematica where they develop mathematics from an abstract version of set theory and get around to proving 1 1 2 on page 362. Really proving that 1 1 2 is trivial. This was put forth in their massive three volume work principia mathematica between 1910 1913. Alfred north whitehead and bertrand russell wrote principia mathematica and published it in three volumes in the years 1910 1913.

He went 100 pages before he finally laid down enough groundwork to define 1 1. 1 1 2 is an accepted supposition. The question says it all.