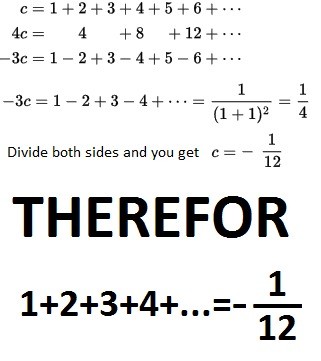
1 2 3 4 5 6 N 1 12 Proof
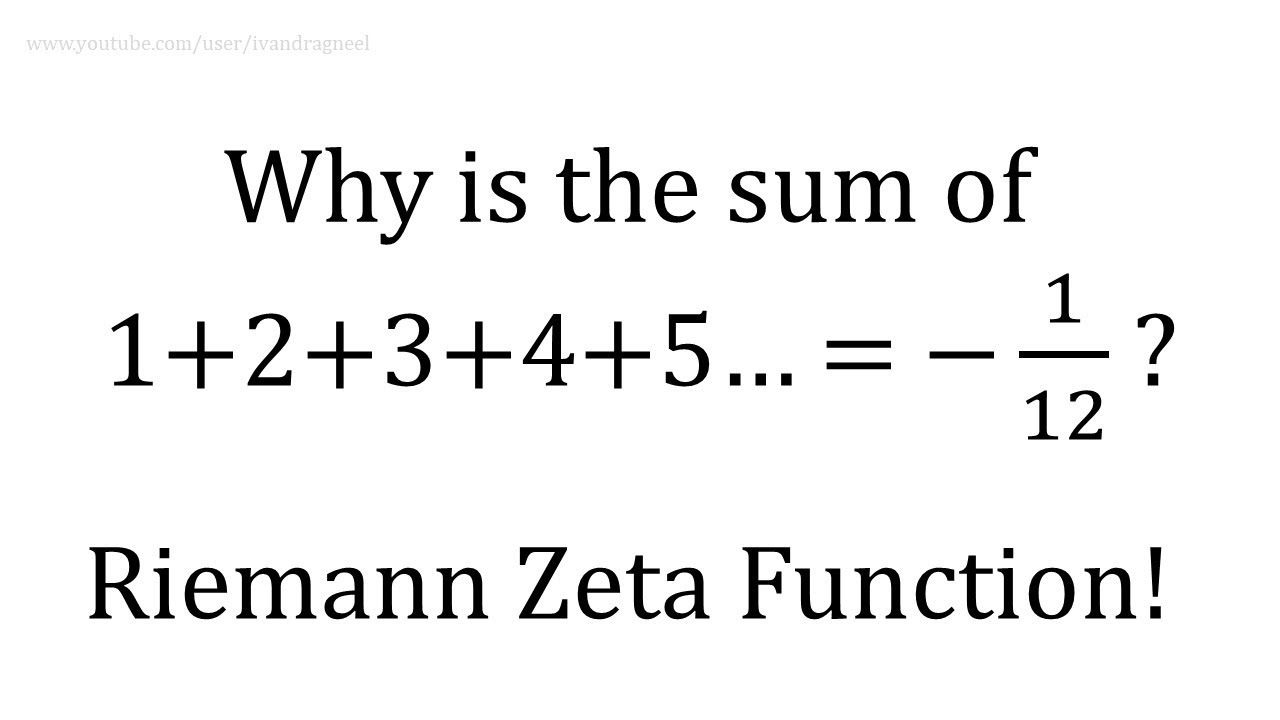
If you watch the first proof posted he points out that they are ignoring the actual boundaries of the parent equation minute 2.
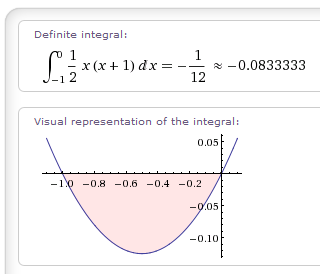
1 2 3 4 5 6 n 1 12 proof. Basically they simplified an equation left out the boundaries and left out the specific conditions s 1 for example. Maths then the answer to this sum is 1 12. C 1 2 3 4 5 6 7 8 4c 0 4 0 8 0 12 0 16 subtracting we get. It says that when you add up all the natural numbers maths 1 2 3 4.
And 1 12 have a relationship with each other but certainly don t equal each other update 2 6 14. The analytic continuation of a function is unique so nailing down ζ s for s 1 is all you need to continue it out into the complex plane. People found the idea so astounding that it even made. Smart news keeping you current the great debate over whether 1 2 3 4 1 12 can the sum of all positive integers 1 12.
A recursive evaluation of zeta of negative integers by luboš motl. The euler maclaurin formula bernoulli numbers the zeta function and real variable analytic continuation by terence tao. That is the series doesn t converge to 1 12 but i am still intrigued by the possibility suggested by the numberphiles that it truly equals 1 12 if all the terms out to infinity are included in the sum. Complex numbers take the form a bi where.
It can sort of. John baez september 19 2008. The only thing about complex numbers you ll need to know here is that complex numbers are pairs of real numbers regular numbers a and b. So i was wrong.
Euler s proof that 1 2 3 1 12 by john baez. The simplest non rigorous proof is due to srinivasa ramanujan and goes roughly as follows. How could i have screwed up so badly. Keep reading to find out how i prove this by proving two equally crazy claims.
1 1 1 1 1 1 1 2. Being a pair of numbers means that complex numbers form the.
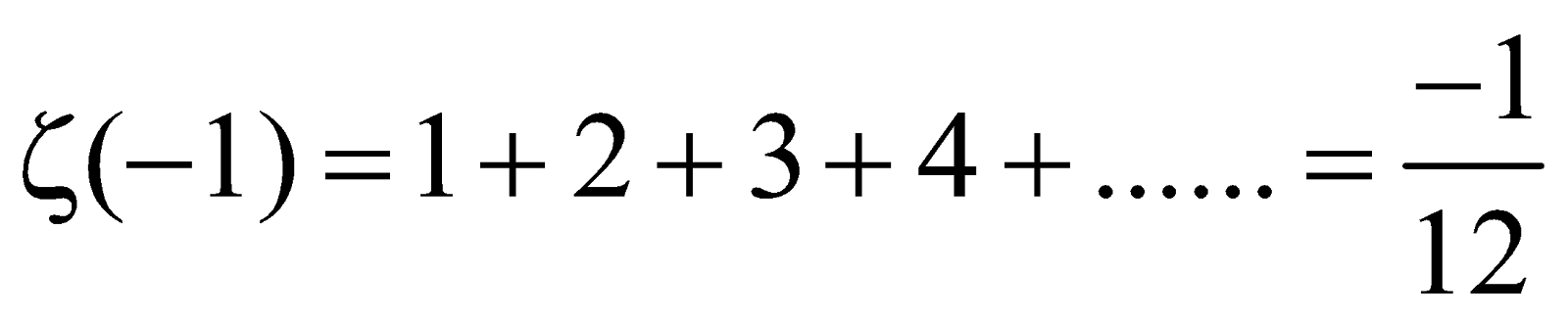


.png)