1 2 2 2 2 3

1 1 3 5 1 1 1 2 2 2 2 3 2 1 1 1 2 1 1 5 1 2 2 2 4 2 2 1 3 2 2 2 2 2 2 3 2 2 2 3 from psy 360 at st.

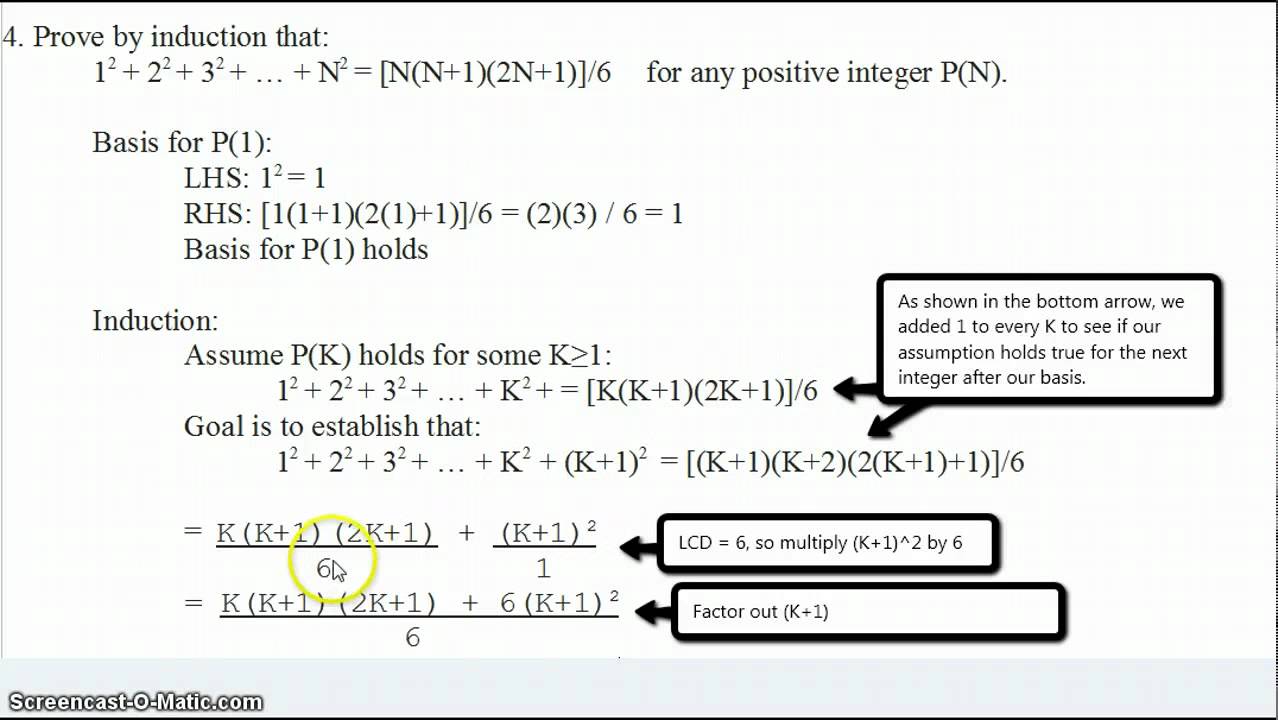
1 2 2 2 2 3. There are two steps in a proof by induction first you need to show that the result is true for the smallest value on n in this case n 1. A 1 2 3 4 5 how to solve the problem. Solutions to exercises on mathematical induction math 1210 instructor. For n 1 the statement reduces to 2 2 22 1 3.

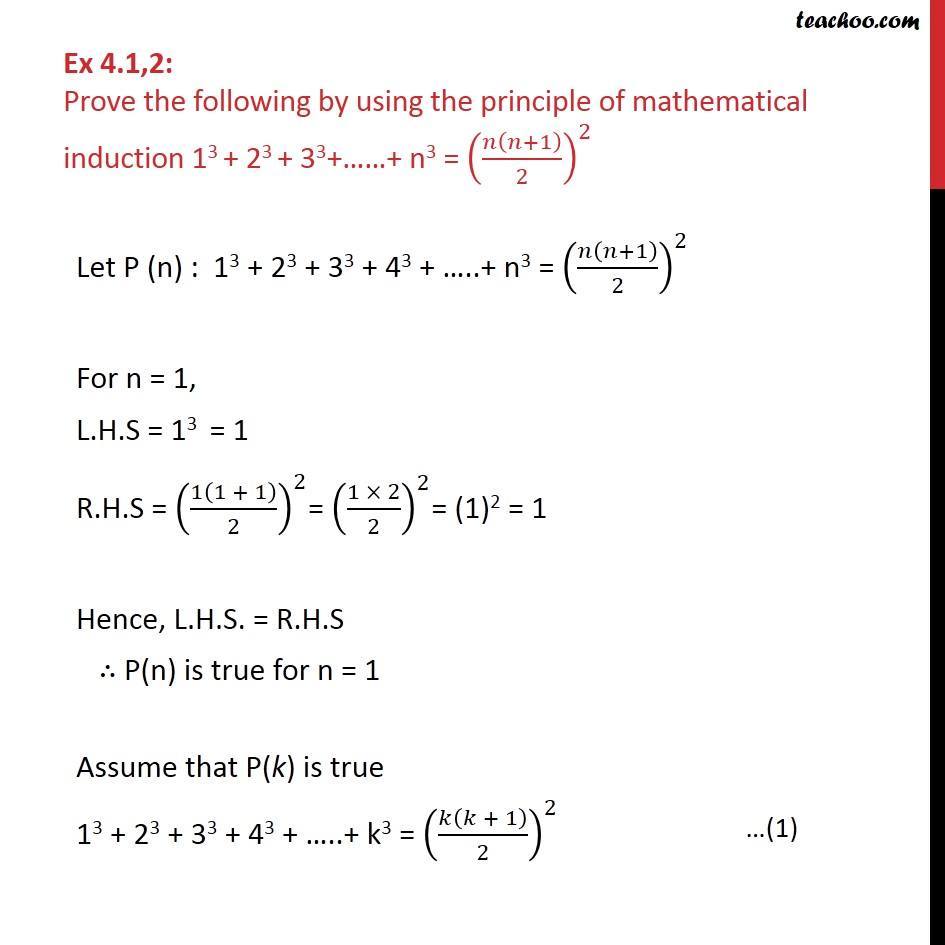
We know that x 1 3 x 3 3x 2 3x 1. Putting x 1 2 n we get. A 1 1 1 1 2 2 2 2 3 3 4 5 5 output b 4 4 2 1 2 also i want to remove the duplicates from a. Por medio del cual se expide el decreto único reglamentario del sector ambiente y desarrollo sostenible.

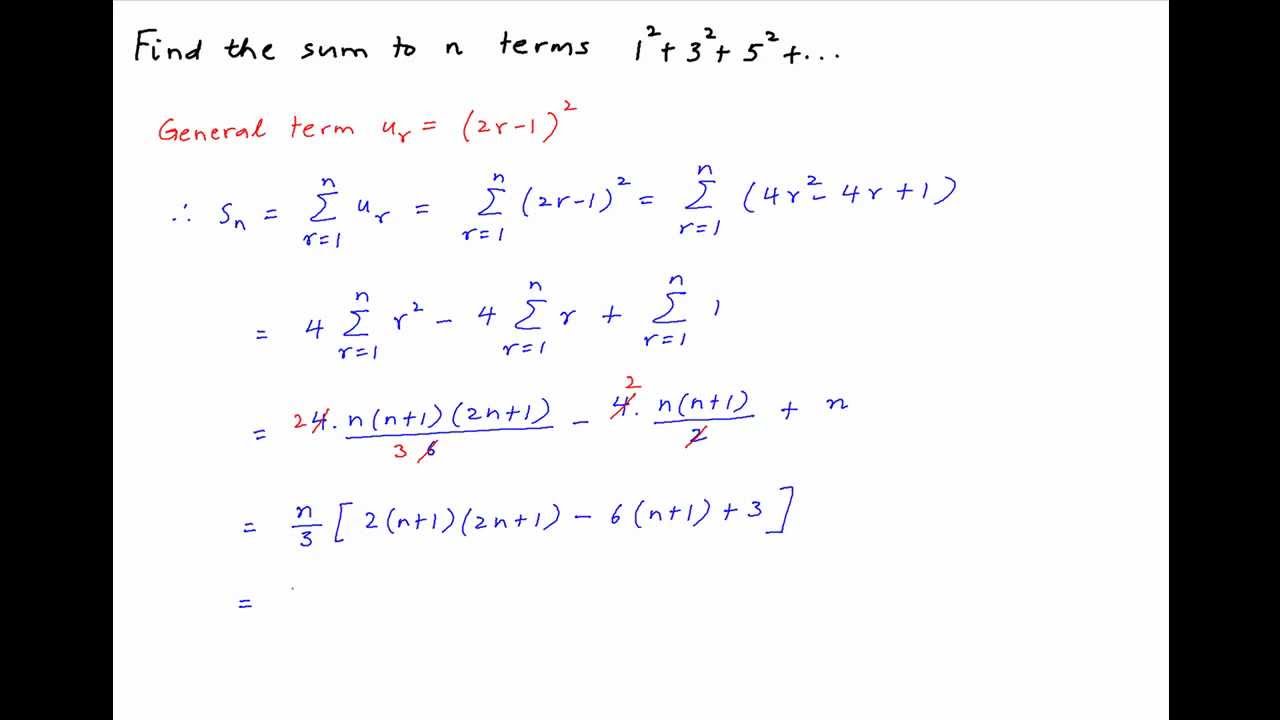
Prove that 1 2 2 2 2 3 2 n 1 2 n 1 for n 1 2 3. Hi zamira i want to state the problem more precisely. In zeta function regularization the series is replaced by the series the latter series is an example of a dirichlet series when the real part of s is greater than 1 the dirichlet series converges and its sum is the riemann zeta function ζ s on the other hand the dirichlet series diverges when the real part of s is less than or equal to 1 so in particular the. 2 23 25 22n 1 2 22n 1 3 proof.

Free math lessons and math homework help from basic math to algebra geometry and beyond. In mathematics the kolakoski sequence sometimes also known as the oldenburger kolakoski sequence is an infinite sequence of symbols 1 2 that is the sequence of run lengths in its own run length encoding and the prototype for an infinite family of related sequences. You can use groupby from itertools package if the list is an ordered list. It is named after the recreational mathematician william kolakoski 1944 97 who described it in 1965 but subsequent.

You should sort the list before using groupby.