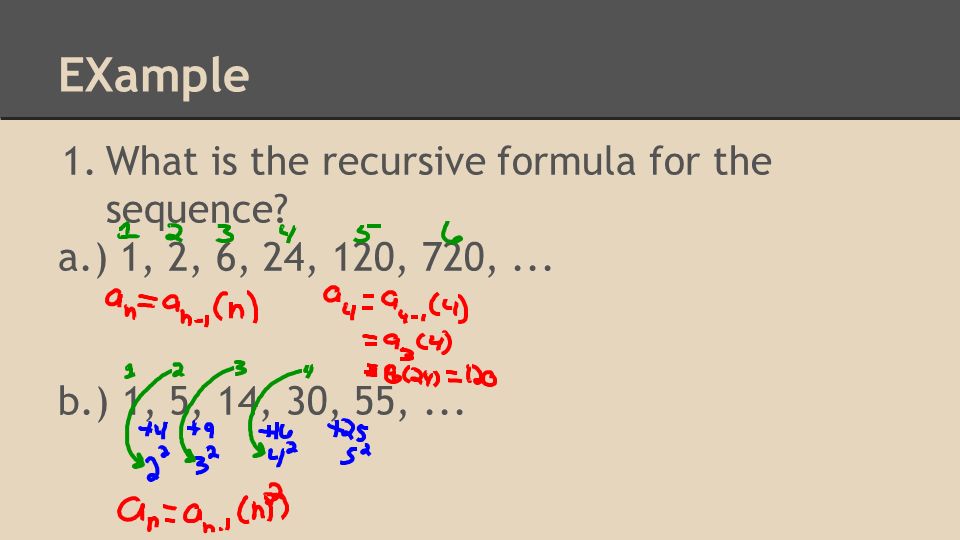
1 1 2 6 24 Sequence Formula

But if we consider only the numbers 6.

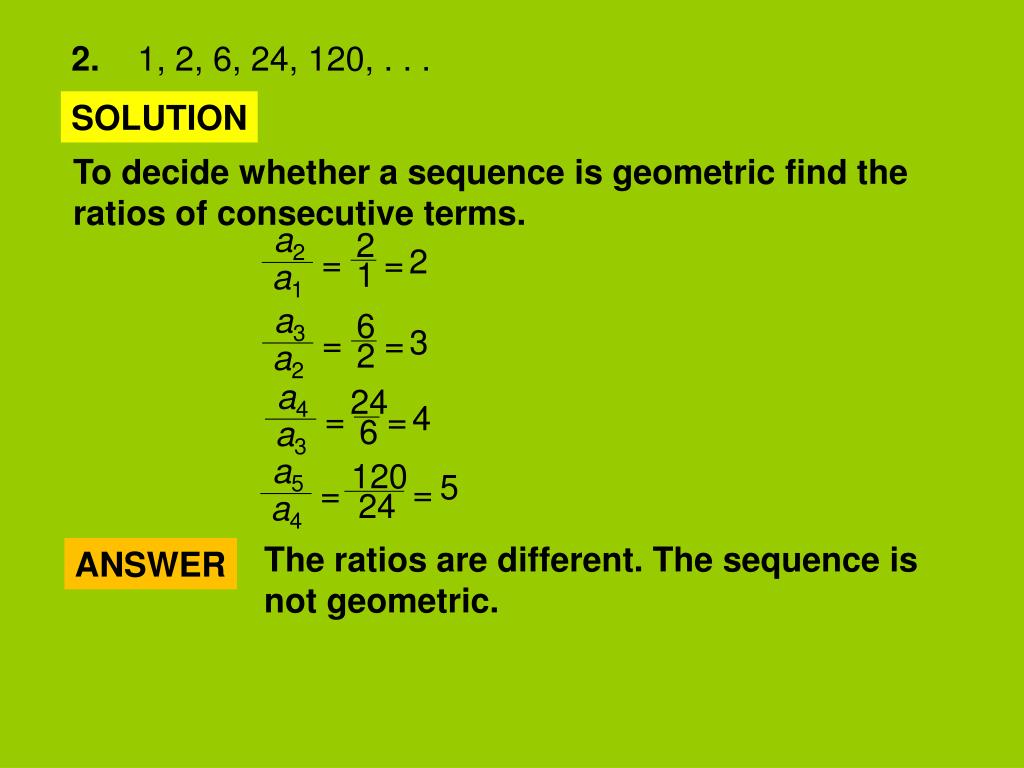
1 1 2 6 24 sequence formula. 1 2 4 8. 2 0 3 8 15 24 n term 1 0 2 3 3 8 4 15 5 24 6 think of another sequence that this one is near. For our example we would write the series as. The sequence is a geometric sequence.

The pattern is 1x1 1 2x1 2 3x2 6 4x6 24 5x24 120 and so on. Find the 125 th term in the arithmetic sequence 4 1 6 11 this arithmetic sequence has the first term a 1 4 and a common difference of 5. F n 1 2 12 24. For example in the sequence 3 6 12 24 48 the gcf is 3 and the lcm would be 48.

Get an answer for 1 1 2 1 6 1 24 1 120 write an expression for the apparent nth term of the sequence. The nth term is equal to the previous term multiplied by n. Not sure of the formula for this pattern. But we can be more efficient than that by using the geometric series formula and playing around with it.

Natural numbers 1 2 3 4 5 6 7 8 9 10 the natural numbers positive integers n ℕ. I hope that this is the answer that you were looking for and it has helped you. Substitute or the 4th term. 1 2 6 24 120 720.

In general the sequence can be expressed. Oeis link name first elements short description a000027. N term 1 1 2 4 3 9 4 16 5 25 6 36 each term of our sequence is 1 less than this sequence and this sequence is easily recognized as the sequence of the squares of n or n. Since we want to find the 125 th term the n value would be n 125.

Let f n 12 and we need to calculate f n 1 which should be 24 if we apply the formula than the answer is. Assume that n begins with 1 and find homework help for other math questions at enotes. The multiplier of the previous number increases by one. The recursive formula for the sequence is.